|
==============================================================================
TOPIC: Neiloid form uncloaked
http://groups.google.com/group/entstrees/browse_thread/thread/ddc004a62f06adef?hl=en
==============================================================================
== 1 of 1 ==
Date: Wed, Dec 12 2007 6:13 pm
From: dbhguru
ENTS,
Gary Beluzo and I are close to completing the article for the next
Bulletin on tree modeling. Will has supplied ample data for the
article and we thank him very much. The article will contain most of
the formulas we have developed to date as part of our continuing
development of the art and science of dendromorphometry, although
more formulas and processes are in the pipeline. One form that I've
had little to say about is the neiloid form and I gave it next to no
space in the article, until Ed pointed out the need to say more
about it. One reason I had avoided the neiloid was that I hadn't had
the basic two-dimensional curve form that gives rise to the volume
formula when rotated around the X or Y axis. So, after Ed's
encouragement, I set about deriving a curve(taper) formula that
produces the volume formula V= (1/4)*pi*r^2. The following summary
shows the curve forms that generate the volume formulas. I should
mentione that there are other neiloid curve formulas. I shoot f
or the most basic. The trees don't know that their supposed to shape
themselves along the line of some exotic taper formula. Much of the
analysis I've seen on the subject, I regard as more academic than
real.
| Let: |
|
|
|
|
R = radius of base of solid |
|
|
|
H = height of solid |
|
|
|
r = radius at point h from vertex of solid |
|
|
h = height at point of r as measured from vertex |
|
|
V = volume of solid |
|
|
|
pi = 3.141593 |
|
|
| |
|
|
|
| Solid |
Curve
Formula |
Volume
Formula |
|
| Cone |
r
= R*(h/H) |
V
= (1/3)*(pi)*r2 |
|
| Paraboloid |
r
= R*SQRT(h/H) |
V=(1/2)*(pi)*r2 |
|
| Neiloid |
r
= R*(h/H)3/2 |
V=(1/4)*(pi)*r2 |
|
| |
|
|
|
The attachment shows the derivation of
the volume formula from the curve formula for the neiloid. I will
include all derivations as attachments in future e-mails.
Bob
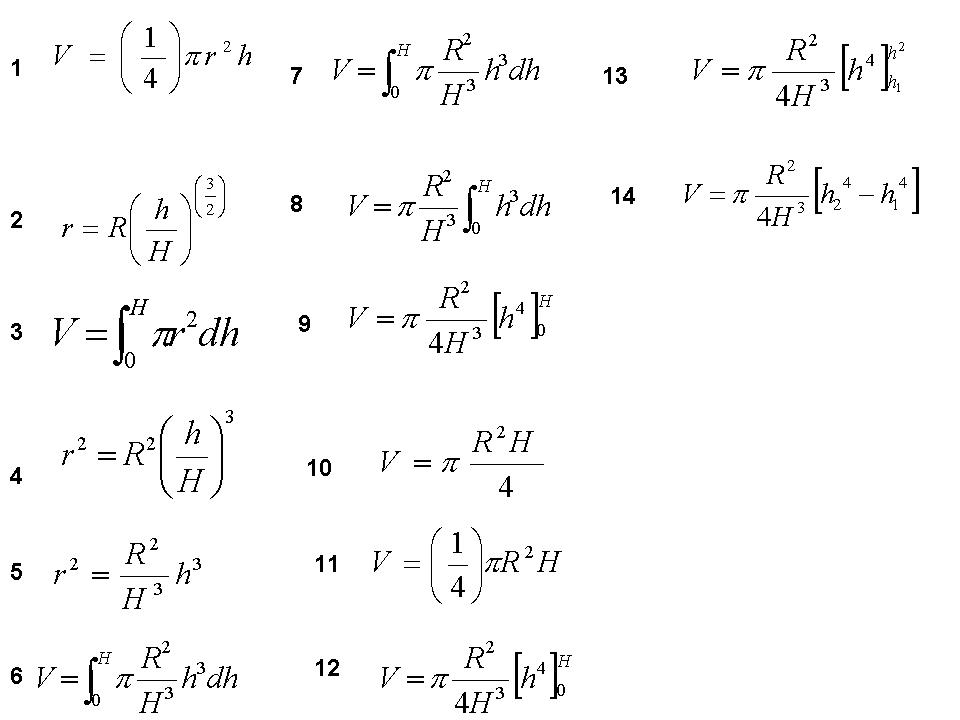
|